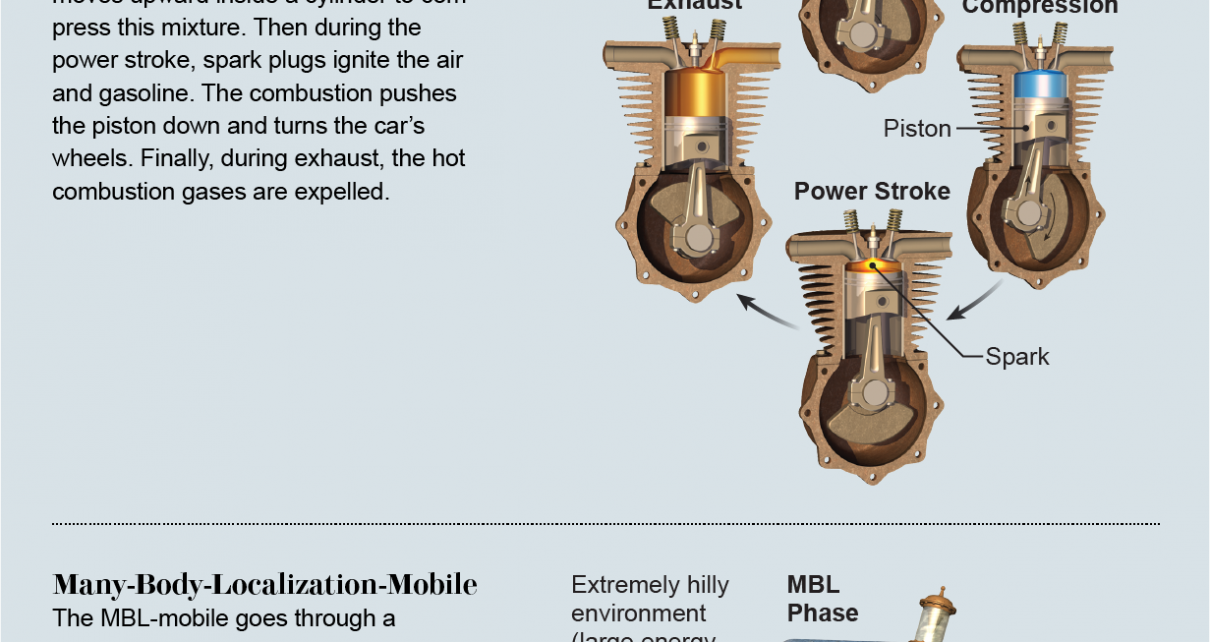
London, at an hour that made Rosalind glad she’d nicked her brother’s black cloak instead of wearing her scarlet one. The factory alongside her had quit belching smoke for the night, but it would start again soon. A noise caused her to draw back against the brick wall. Glancing up, she gasped. An oblong hulk was drifting across the sky. The darkness obscured the details, but she didn’t need to see; a brass-colored lock would be painted across the side. Mellator had launched his dirigible.
Welcome to steampunk. This genre has expanded across literature, art and film over the past several decades. Its stories tend to take place near nascent factories and in grimy cities, in Industrial Age England and the Wild West—in real-life settings where technologies were burgeoning. Yet steampunk characters extend these inventions into futuristic technologies, including automata and time machines. The juxtaposition of old and new creates an atmosphere of romanticism and adventure. Little wonder that steampunk fans buy top hats and petticoats, adorn themselves in brass and glass, and flock to steampunk conventions.
These fans dream the adventure. But physicists today who work at the intersection of three fields—quantum physics, information theory and thermodynamics—live it. Just as steampunk blends science-fiction technology with Victorian style, a modern field of physics that I call “quantum steampunk” unites 21st-century technology with 19th-century scientific principles.
Our goal is to update the laws of thermodynamics—the study of work, heat and efficiency—to meet the demands of cutting-edge experiments, technologies and theory. Thermodynamics was born when steam engines drove the Industrial Revolution. But as technology shrinks, thermodynamics and information couple in smaller and smaller systems. The spotlight has swept from trains to nanoscale engines, living cells’ molecular motors and the smallest possible refrigerators. We must now investigate how to apply traditional thermodynamic concepts such as heat, work and equilibration to modern quantum systems.
Victorian physics meets millennial science
By 1800 Thomas Savery and Thomas Newcomen had invented, and James Watt and Matthew Boulton had refined, the steam engine. Thinkers then wondered how efficiently such engines could pump water out of mines. Their studies grew from practicalities to questions of fundamental physics, such as why time flows only in one direction. The field of thermodynamics is grounded in this work.
This branch of physics describes many-particle systems, such as steam, in terms of large-scale properties, such as temperature, pressure, volume and energy. Energy in transit falls into two classes, work and heat. Work is well-organized energy usable for a purpose, like turning a mill wheel. Heat is the energy of random motion—of particles jiggling.
Thermodynamicists quantify randomness with a number called entropy. Every particle in a canister of steam has a position and a momentum (the particle’s mass times its velocity). The set of all the particles’ positions and momenta we call the steam’s microstate. We cannot know the microstate, because the canister contains about 1024 (1 followed by 24 zeroes) particles. Imagine trying to locate them all! Instead we track the probability that the steam occupies this or that microstate. Entropy quantifies our uncertainty. According to the second law of thermodynamics, the entropy of a closed, isolated system cannot shrink. This fact underlies the reality that time flows in a single direction.
But the steam engines central to traditional thermodynamics resemble today’s technologies about as much as top hats resemble virtual-reality headsets. Many modern inventions and experiments involve small, complex quantum systems. Quantum theory is the physics of atoms, electrons and other constituents of matter. They can behave in ways impossible for larger, classical systems, such as steam canisters, factories and people. For instance, quantum particles can share entanglement, a type of ultrastrong correlation. If you entangle two atoms and measure one, the other atom changes instantaneously, even if it is across a continent. Physicists can use entanglement to process information in ways impossible with classical systems. The study of how we can solve computational problems, communicate, secure information and enhance measurements with quantum systems is called quantum information theory. This theory is a useful mathematical tool kit for implementing our update to thermodynamics. How do the two fields connect? To reason about information, we have to confront ignorance. Information theorists quantify ignorance with entropy, just as thermodynamicists do.
Quantum computers, for instance, are systems where both quantum information theory and thermodynamics are key. Google, IBM and other institutions are hard at work building such machines, which aim to break certain encryption schemes and to model certain materials far more quickly than any classical computer. Most quantum-computing systems need to be cooled to a temperature near absolute zero. Cooling amounts to dissipating heat, a thermodynamic quantity. Yet quantum computers look nothing like the engines for which thermodynamics was developed.
Efforts to apply thermodynamic concepts to quantum settings date to the mid-20th century, when Joseph Geusic, E. O. Schulz-DuBois and H. E. Derrick Scovil proposed the first quantum engine.It was made from a maser, which operates like a laser but releases microwave light. Later, Ronnie Kosloff of Hebrew University of Jerusalem and his colleagues helped to turn quantum engines into their own subfield. Another pioneer is Marlan Scully, sometimes called the “quantum cowboy,” who works on quantum optics at Princeton University and Texas A&M University and also raises cattle. Meanwhile theorists Gian Paolo Beretta, the late Elias Gyftopoulos and the late George Hatsopoulos studied the arrow of time from a quantum perspective. And a seminal publication was Seth Lloyd’s 1988 Ph.D. thesis at the Rockefeller University, “Black Holes, Demons, and the Loss of Coherence: How Complex Systems Get Information, and What They Do with It,” which established many important ideas for the field of quantum thermodynamics.
Quantum Steampunk Tools
As we have seen, entropy plays an important role in thermodynamics, information theory and quantum theory. Entropy is often thought of as a single entity, but in fact, many breeds of entropy exist in the form of different mathematical functions that describe different situations. The best-known breeds were introduced into thermodynamics by Ludwig Boltzmann and Josiah Willard Gibbs during the 1800s, into information theory by Bell Telephone Labs employee Claude Shannon in 1948, and into quantum information theory by theoretical physicist John von Neumann in 1932. These entropies quantify not only uncertainty but also the efficiency with which we can perform information-processing tasks, like data compression, and thermodynamic tasks, like the powering of a car.
Identifying new entropy functions for modern, small-scale quantum systems is one of the key tasks of quantum steampunk theorists. Suppose we are trying to use entanglement to share information in a certain channel. We might ask, Is there a theoretical limit to how efficiently we can perform this task? The answer will likely depend on an entropy.
Another quantum steampunk goal is building what physicists call resource theories. These theories highlight the constraints under which we operate. For instance, the first law of thermodynamics constrains us to conserve energy: We cannot create or destroy energy; we can only shunt it from one form and one system to another. Physicists might find a situation in which there is a constraint, such as an environment with a fixed temperature, and then try to model the situation mathematically with a resource theory. Using the resource theory, we can calculate the optimal efficiency with which a task can be performed. Typically the efficiency equals a function of an entropy.
A third area of focus in our quest to update thermodynamics is to derive equations called fluctuation relations. These equations are extensions of the second law of thermodynamics, which dictates that the entropy in a closed, isolated system cannot decrease. Fluctuation relations govern small systems subjected to strong forces and tell us about the work those forces perform.
In 1996 Christopher Jarzynski, now at the University of Maryland, proved one of the best-known fluctuation relations. Thermodynamicists call it Jarzynski’s equality, although Jarzynski is so modest, he never does. Experimentalists use this equality to measure a certain thermodynamic property of small systems. As an example, imagine a DNA strand floating in water, with the same temperature as its surroundings. The strand has some amount of free energy, which is basically the energy that a system can draw on to perform work. Using lasers, scientists can trap one end of the strand and pull the other end. After they hold the strand taut for a while, the DNA will return to the solution’s temperature, at which point the strand will have a different amount of free energy. The difference in free energies has applications in chemistry, pharmacology and biology. We can estimate the free-energy difference by stretching the strand in many trials, measuring the work required in each trial, plugging our data into Jarzynski’s equality and solving the equation.
How many trials must we perform, Jarzynski and I asked, to estimate the free-energy difference with a certain precision? We calculated the minimum number of trials that one would likely have to perform and proposed a scheme for quantifying the precision, using small-scale information theory. In other recent work, my collaborators and I showed that fluctuation relations and newfangled entropy functions are two consistent approaches to small-scale thermodynamics, and we used each approach to elucidate the other. Quantum thermodynamicists in London, Cologne, and elsewhere have extended and sharpened this research.
A New Quantum Engine
Just as traditional thermodynamics helped to describe the physics of steam engines, our efforts in quantum thermodynamics can help us invent quantum engines. Experimentalists have now created quantum engines with photons (particles of light), electronic systems and superconducting qubits (quantum circuits in which current can flow forever without dissipating).
Recently I designed a new quantum engine with Christopher D. White, now at the University of Maryland, Sarang Gopalakrishnan, now at the City University of New York, and Gil Refael of the California Institute of Technology. Being theorists, we initially devised the engine as a thought experiment that existed in our minds. But we are also envisioning how scientists could build a real version of the engine using the quantum tools found in laboratories today. For instance, by cooling atoms, then trapping and manipulating them with lasers, one could bring our design to life.
Our engine involves a phase of matter called many-body localization (MBL)—a variation on the more familiar phases liquid, solid and gas. Quantum particles can be in this phase if they repel one another and can hop slowly around a rough, steep, random landscape. A key element of an MBL system is its “athermality”: It is not in thermal equilibrium. Particles in thermal equilibrium explore the available space quickly and randomly. If you let steam explore for a long time, large-scale properties such as the temperature and volume will settle down and quit changing much.
But MBL particles stay in one area rather than moving around, in contrast with steam particles. A lack of thermal equilibrium serves as a resource in thermodynamic tasks. Car engines, for instance, rely on having a hot fluid near a cold fluid. The pair of fluids is not at thermal equilibrium, because the hot particles are localized in one region and the cold particles in another—no particle explores the whole space. As a car engine takes advantage of the fluids’ athermality, my collaborators and I took advantage of MBL particles’ athermality. We call our construction the MBL-mobile.
A car engine undergoes four steps that form a cycle, or a closed loop. By the end of the loop, the engine returns to its initial state, having propelled the car some distance by transferring heat from the hot fluid to the cold. The MBL-mobile, too, undergoes a four-step cycle. In our engine cycle, we ratchet, or transition, the atoms from a thermal phase, in which particles can spread throughout the space, to MBL and back. To ratchet the engine, we change the landscape the particles inhabit from fairly flat to rough by manipulating the lasers’ settings. Before each ratcheting, the engine exchanges heat with an external environment. The engine interacts with a hot environment when in its thermal phase and with a cold environment when in its MBL phase. In summary, the four steps are: (1) exchange heat with a hot environment in the thermal phase, (2) ratchet from the thermal phase to MBL, (3) exchange heat with a cold bath and (4) ratchet from MBL to the thermal phase.
We assessed how well an MBL-mobile could work by calculating its power and efficiency and comparing them with those of other engines. For instance, some bacteria have flagella, or long, whippy tails rotated by motors. How do these small engines compare with ours? Our engine, we estimated, can output about 10 times a flagellum’s power. On the other hand, how does our quantum engine compare with a car’s engine? We estimated the two engines’ power densities, or the power output per unit volume: a car engine uses space more effectively, though only about 10 times more.
The MBL phase gives our engine four advantages. First, the engine can have any size, from 10 particles to infinitely many. To build a large engine, you start with a mini engine of 10 particles. You build many copies of the mini engine and then operate them side by side. If the mini engines behaved thermally, they would interfere with one another because one mini engine’s particles would stray into another mini engine. MBL ensures that what happens in one mini engine stays there. Thus, you can cram many mini engines close together, giving the entire engine a high power density: the MBL-mobile’s second advantage.
The third advantage surfaces if you run the engine in many trials. In some trials, the engine will perform work. In a few trials, though, the engine will absorb work, doing the opposite of what it should. Fewer of these worst-case trials occur if you ratchet the engine between MBL and thermal phases than if you ratchet the engine around within the MBL phase. Moreover, the amount of work varies less from successful trial to successful trial if you take advantage of MBL; MBL enhances the engine’s reliability.
Our success with the MBL-mobile, at least in thought experiments, suggests that MBL may have more applications in other thermodynamic tasks that need undertaking. For example, imagine reversing our cycle. The engine should refrigerate, transferring heat from the cold environment to the hot. Quantum systems require refrigeration for properties such as entanglement to manifest. An MBL refrigerator could serve to cool many-particle quantum systems. Alternatively, scientists also wrote a proposal to use MBL to store energy. And recently, along with my collaborators, I have begun trying to create a real-life version of the engine using another set of tools: superconducting quantum bits set in a magnetic field. Opportunities abound when we apply quantum steampunk thinking to materials science.
Gazing through a quantum monocle
A steampunker gazes into the future through a monocle. What does she see? A mathematical and physical tool kit is solidifying at the intersection of quantum theory, information theory and thermodynamics. We are also working to apply that tool kit to other spheres of science: materials science, as in the MBL-mobile; chemistry; high-energy physics, such as black holes and the fabric of spacetime; and atomic, molecular and optical physics.
Technologies cry out for applications. Most quantum steampunk work is theoretical, although real-world experiments have begun and are multiplying. But just as the development of thermodynamics helped to drive the Industrial Revolution, new inventions should follow from quantum, small-scale and information thermodynamics. MBL engines will not power our cars this decade. But molecular switches, solar-fuel harvesters and heat-dissipating transistors are small-scale technologies tied to thermodynamics. They should guide theory.
Another challenge is to unify the different efforts within quantum steampunk—newfangled entropies, resource theories, fluctuation relations, quantum-thermal machines, and more. These are just some of the many different kinds of work going on around the world and new tools being developed. Reconciling these realms’ different definitions and results will solidify a theory for quantum thermodynamics.
Thermodynamics carries the whiff of engine grease and grit, of steaming across the countryside in the first trains and conquering the waves in the first ocean liners, of marveling at the landscape from a hot-air balloon. Quantum information science is transforming how we understand computation, communication, cryptography and measurement. You are reading about this confluence of old and new in Scientific American, but you might as well be holding a novel by H. G. Wells or Jules Verne.